STRENGTH of a solid body, the ability to maintain continuity, integrity, configuration, dimensions, ability to elastic deformation under the influence of external loads for a certain time. nature (mechanical, thermal, electrical, magnetic, etc.). In the narrow sense, P. is mechanical. the property of real solids to resist destruction. The concept of rigidity plays a significant role in fundamental (solid state physics, continuum and deformable solid mechanics) and applied (strength of materials, soil mechanics, materials science, etc.) sciences.
According to the nature of the temporary change created by external loads, loads are distinguished: stationary, quasi-stationary, low-cycle (acting periodically with the number of cycles <104), cyclic (number of cycles from 104 to 105), high-cycle (number of cycles >105), dynamic and shock. Various types also have corresponding names. P. types: static, dynamic, shock (strain rate 10–4–10–7 s), low- and high-cycle fatigue, etc. The type of external load (for example, thermal strength) is also added to the P. characteristics.
Physical nature of strength
The power of solids is caused by the forces of interaction between the atoms and ions that make up the body. These forces depend on ch. arr. on the relative arrangement of atoms. For example, the strength of interaction between two neighboring atoms (if we neglect the influence of surrounding atoms) depends only on the distance between them. At the equilibrium distance (approx. 10 nm) this force is zero. At smaller distances the force is positive and the atoms repel, at larger distances they attract. At some critical point. at a distance $r_{cr}$ the force of attraction is maximum in absolute value and equal to $F_t$. For example, if, when stretched, a cylindrical of a rod with cross section $S$, the acting force (load) $\boldsymbol P$, directed along its axis, is such that the external force exerted on a given pair of atoms exceeds the max. force of attraction $F_т$, then the atoms move away from each other without hindrance. However, for a body to collapse along a certain surface, it is necessary that all pairs of atoms located on both sides of the surface in question experience a force exceeding $F_t$. The voltage corresponding to the force $F_т$ is called theoretical. tensile strength $σ_т$ ($σ_т≈0.1E$, where $E$ is Young's modulus). However, in practice, destruction is observed under a load $P^*$, which corresponds to a stress $σ=P^*/S$, 100–1000 times less than $σ_t$. The discrepancy between the theoretical Real load is explained by inhomogeneities in the structure of the body (grain boundaries in a polycrystalline material, foreign inclusions, structural defects, etc.), due to which the load is distributed unevenly over the cross section of the body.
If on a small surface area (but significantly larger than the cross section of one atom) the local stress is greater than $σ_т$, a rupture will occur along this area. The edges of the rupture will diverge to a distance greater than $r_{cr}$, at which the interatomic forces are already small, and a microcrack will form. The nucleation of microcracks at stresses below $σ_т$ is promoted by thermal. fluctuations. Local stresses are especially high at the edge of the formed crack, where stress concentration occurs, and the larger the crack size, the greater they are. If this size is greater than a certain critical one, a stress exceeding σт acts on the atoms at the edge of the crack, and the crack grows further along the entire cross-section of the body, and destruction occurs.
Methods for determining the strength of a structural material
Conducting static strength tests is testing template samples of a certain shape. Based on the results of the experiments, experts draw a diagram on which you can clearly see how the material deforms under stress. Graphic data helps to evaluate the elastic and yield limits, and temporary resistance. To determine the parameters of a particular material, special calculations are carried out to calculate the fatigue load and ultimate stress.
Methods for determining the strength of a material depend on its variety and type of building structure. For example, a standard way to evaluate the performance of a brick is to test the compression of two whole bricks that are stacked on top of each other. Ultrasonic techniques are used to study sand-lime brick.
All research methods can be divided into two large groups - destructive and non-destructive testing. They apply to individual building structures, specimens and individual elements.
Whenever possible, experts try to give preference to non-destructive testing methods that do not require dismantling and disassembling the structure. Although samples are taken from the least important functional elements, standard strength testing methods affect the stability and reliability of the building. But it is not always and not possible to assess the strength of all construction products using non-destructive testing methods.
Destructive testing methods
A distinctive feature of this type of research is testing on control samples until they are completely destroyed. For example, a brick can be compressed or otherwise influenced from the outside until it cracks or crumbles. To do this, part of the material is removed from the structure and sent to the laboratory to evaluate the strength characteristics.
To determine the sampling site, the accessibility of the sample, the degree of load and damage, and the intensity of operation of the building structure are taken into account. Destructive testing methods make it possible to calculate the physical properties of the sample with minimal error. But they require serious labor. The main disadvantage of destructive testing is the need to violate the integrity of the building. This is not always possible, so experts try to evaluate the characteristics of building materials using non-destructive testing.
Non-destructive testing methods
Research using non-destructive methods is actively used in the technical examination of residential, industrial, administrative buildings and structures, objects of historical and cultural heritage. They can be based on various technologies:
- mechanical: the elastic rebound method, the study of plastic deformations and impact impulse are often used to examine concrete;
- radiation: methods are based on the use of radioisotopes and neutrons;
- magnetic: methods of magnetic particle and induction assessment;
- acoustic: research by exposure to ultrasound, assessment of the effects of acoustic emission;
- radio wave: study of the distribution of waves of different lengths in a material;
- electrical: determination of characteristics through calculation of electrical resistance, electrical inductance and electrical capacity of a building material.
With the help of modern instruments and technology, it is possible to determine the strength characteristics of a product without design changes and maintain the original physical and mechanical parameters of the materials.
Strength theories
In structures under loading, as a rule, a complex stress state arises, characterized at each point by three chapters. stresses $σ_1,\,σ_2,\,σ_3$ (numbering is determined by the conditions $σ_1⩾σ_2⩾σ_3$). For calculations on P., P. theories (criteria) are used—formalized relationships that link a certain combination of chapters. stresses $f(σ_1,σ_2,σ_3)$ with a dangerous stress $σ_{op}$, at which destruction begins (tensile strength), determined from experiments on uniaxial loading.
In the first theory of P. (the theory of max. normal stresses) it is assumed that failure under a complex stress state occurs when the max. Ch. voltage $σ_1$ is equal to dangerous voltage: $σ_1=σ_{op}$. This theory does not take into account the influence of the second (and in the case of a triaxial stress state, the third) Ch. voltage, but it agrees well with experiment. data for brittle materials, when the first ch. the voltage is much greater than the other two.
The basis of the second theory of P. (the theory of maximum linear deformations) is the assumption that destruction occurs when the maximum is reached. linear deformation of dangerous value: $ε_1=ε_{op}$. The deformation εop can be found from Hooke’s law: εop=σop/E, where $E$ is the elastic modulus (Young’s modulus). Taking into account the generalized Hooke's law, the destruction condition corresponding to the second theory of P. has the form: $σ_1-ν(σ_2+σ_3)=σ_{op}$, where $ν$ is the coefficient. Poisson. This theory is well confirmed for brittle materials in triaxial compression experiments.
The third theory of P. (the theory of max. tangential stresses) is based on the hypothesis that the cause of destruction is max. tangential stresses $τ_{max}$. The destruction condition in this theory of P.: $σ_1-σ_3=σ_{op}$.
According to energy theory of P., the condition of P. has the form 1√2√(σ1−σ2)2+(σ2−σ3)2+(σ3−σ1)2=σopThird theory and energy. P.'s theory is well confirmed in experiments with plastic materials.
For materials that have different resistance to tension and compression, Mohr's theory of strength is often used, in which the P. condition is determined by the equality $σ_1-kσ_3=σ_{op}^{(p)}$, where $k=σ_{op}^{ (p)}/σ_{op}^{(c)}$, $σ_{op}^{(p)}$ – dangerous stress in tension, $σ_{op}^{(c)}$ – in compression .
With the development of industrial and transport engineering, construction of complex engineering. structures, there was a need for other P. criteria. P. theories were developed that related stress, deformation, time and temperature. This is the Hubert-Henki-Mises theory, which takes into account the energy of shape change of nonlinear elastic deformations, the generalized octahedral theory. stresses, etc. In 1933, S. N. Zhurkov and A. P. Aleksandrov created molecular kinetics. P.'s theory, based on thermofluctuation physical. the nature of elementary acts of interatomic and intermolecular rearrangements and the activating influence of mechanical. stress. In the 20th century During the construction of aircraft, pipelines, etc., it turned out to be necessary to develop performance criteria that take into account the processes of damage accumulation. Based on solutions to problems on stress concentration in a body with cuts and slits in the 1960s. The theory of cracks was developed and damage parameters were introduced. scale, which were used by A. A. Ilyushin when creating theoretical. fundamentals of kinetic theory by P. and Yu. N. Rabotnov in its practical work. application.
Strength is the ability of materials to resist internal stresses arising under the influence of external loads.In structures, building materials, depending on the nature of the load, can experience various stresses: compression, tension, bending, etc.
This property of building materials is characterized by tensile strength, i.e., the stress in the material corresponding to the load at which the sample fails. The ultimate compressive or tensile strength (R) is equal to the ratio of the destructive force (Rbreak) to the original area of the sample (F):
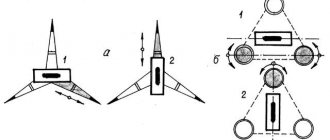
The destructive, i.e. maximum, load is determined using hydraulic presses or tensile testing machines of various capacities. The results of these tests depend on the strength of the substance from which the material is composed, its shape, size, rate of load increase, etc. For tensile testing, samples in the form of eights are usually used, and for compression - cubes of various sizes (with edge lengths from 3 to 30 cm) or cylinders. When testing cylinders, the compressive strength values are approximately 25% less than those of cubes. Small samples have higher compressive strength than large ones. This is explained by the fact that compression is accompanied by transverse expansion. The two planes of the test sample are pressed against the press plates, and the resulting frictional forces keep the adjacent parts from lateral expansion and, therefore, from destruction. The greater the relative height of the sample, the less the influence of the supports of the press plates on its strength. For this reason, most stone materials fail when compressed as shown in Figure 2.
Changes in compressive strength for stone materials depending on the size of the sample are given in Table 1.
Rice. 2. The nature of the destruction of a cube made of stone materials when tested in compression.
Table 1 Transition coefficients for determining the compressive strength of stone materials
Stone materials (rocks, concrete, brick) in tension can withstand a load that is 10-20 times less than in compression. Other building materials, such as steel, wood, and plastics, resist both compression and tension equally well.
The flexural strength is determined by testing small beams made from the material being tested. These beams are destroyed with one or two concentrated loads (Fig. 3).
Rice. 3. Schemes for testing building materials for bending with concentrated loads: a - one; b - two.
The bending strength is equal to: with one load
with two loads
where Рbreak is the breaking load, kg; l — distance between supports, cm; a is the distance between the loads, cm; b and h are the width and height of the beam, respectively, cm.
The conditions for carrying out these tests are given in the standards (GOST) for the relevant materials.
However, it must be borne in mind that various structures and structures are calculated not by their ultimate strength, but by their permissible stress:
where z is the safety factor, the value of which is more than one. This requirement for the permissible voltage is determined by the following reasons.
1. The indicators obtained during testing give an idea only of the average strength of materials. Due to their heterogeneity, materials in the weakest places are destroyed before the stress reaches this average value. Therefore, the greater the heterogeneity of the material, the greater the safety factor.
2. Many materials loaded to the point of stress reaching 50-70% of the ultimate strength are severely deformed.
3. With repeated variable loads, the so-called fatigue of materials occurs, and they can fail at a stress equal to half the tensile strength.
4. The action of various atmospheric factors causes a change in the initial properties of materials, and they age, which is naturally accompanied by a decrease in their strength.
To ensure sufficient strength of structures under the influence of the listed factors, as well as loads not taken into account in the calculations or not taken into account accurately enough due to imperfect test methods, the building design standards establish certain safety margins for different materials and structures (z = 2-3 or more) .
Two important properties of building materials - volumetric weight and strength - require the introduction of another coefficient - structural quality (K.K.K.). It is characterized by the ratio of the strength of the material to its volumetric weight:
The best material in terms of construction will be the one that has the highest coefficient of structural quality. Such materials make it possible to create durable and at the same time lightweight structures. K.K.K. of basic building materials has the following values:
Contemporary issues
The solution of current problems of P. is associated with the development of phenomenological. global criteria for P. in bi- and triaxial stress-strain states in areas with different signs of Ch. stresses and deformations when applying a variable load to a static one, especially in areas of high stress concentration. The following are considered: problems of calculating the load under irregular and complex conditions of repeated loading, including contact alternating stress state; fatigue at high and low temperatures, as well as under pulsed loads (pulse metal processing); establishing P. conditions with a non-uniform distribution of mechanical components. properties of structural elements made of reinforced fibrous materials, conditions of thermal strength of refractory materials (tungsten, molybdenum, niobium, tantalum, various alloys based on them). Theories and methods for calculating structural sensitivity are being developed. properties of materials (fragile P., accumulation of damage associated with loosening, destruction of the structure, long-term P., fatigue at medium and small vibration amplitudes). Solving problems in the design of mine workings and calculating the seismic resistance of structures and structures are associated with the development of the theory of tectonics. processes and phenomena taking into account rheological properties and features of the propagation of disturbances in soils and real patterns of occurrence of layers in the earth's crust.
Order concrete strength determination
Our organization determines the strength of concrete of any building structures using both non-destructive and destructive methods.
To determine the cost and receive detailed advice on all your questions, you can call +7 (495) 128-53-66 or leave a request using the form below, and we will call you back.
We guarantee a commercial offer within 24 hours.
Licenses and Certificates
Certificate of conformity
Extract from the register of SRO JV
Extract from the register of SRO JV – page 2
Extract from the register of SRO LI
General requirements for structural materials
Structural materials are materials intended for the manufacture of machine parts and engineering structures that are subject to mechanical loads.
Machine parts are characterized by a wide variety of shapes and sizes, as well as different operating conditions. They operate under static, cyclic and shock loads, at low and high temperatures, in contact with various media. These factors determine the requirements for structural materials, the main of which are operational, technological and economic. Performance requirements are of paramount importance. In order to ensure the functionality of specific machines and devices, the material must have high structural strength.
Structural strength is a set of mechanical properties that ensure reliable and long-term operation of a material under given operating conditions.
Operating conditions are determined by the working environment (liquid, gaseous, ionized, radiation, etc.), which can have a negative impact on the mechanical properties of the material. As a result of chemical and thermal effects, it can cause damage to the surface due to corrosion cracking, oxidation, scale formation, etc. In order to avoid the negative effects of the working environment, the material must have not only mechanical, but the required physical and chemical properties - corrosion resistance, heat resistance , cold resistance, etc.
The temperature range of operation of modern materials is very wide, therefore, to ensure performance at high temperatures, the material requires heat resistance, and at low temperatures, cold resistance.
Technological requirements are aimed at ensuring the least labor intensity and ease of manufacturing parts and structures. They are assessed by the manufacturability of the material, determined by good machinability, pressure, weldability, castability, as well as the required hardenability and the absence of deformation and warping during heat treatment. The manufacturability of the material ultimately determines the productivity and quality of parts manufacturing.
Economic requirements - the material must be low cost and accessible. For example, steels and alloys must contain a minimum amount of expensive alloying elements. Moreover, their use must be justified by a corresponding increase in the performance properties of the parts.
Dynamic strength under cyclic loads
A characteristic of dynamic strength under repeatedly variable loads is the tensile strength (endurance, fatigue limit) of a material, the value of which is less than the value of static strength.
Tensile strength
The maximum absolute value of the cycle stress at which fatigue failure of the material does not yet occur for a given number of load cycles.
The magnitude of the tensile strength depends on a number of factors:
• type, structure and defects of the material, • manufacturing and processing technology, • testing environment and temperature, • surface condition, • stress concentration, • sample dimensions, • load mode, etc.
The tensile strength can vary widely - under the most unfavorable conditions it can decrease by 5-10 times compared to the tensile strength of the material under normal conditions. These changes cause significant difficulties in the design of machines and structures due to the need to eliminate their fatigue failures.
For each case of operating conditions, the tensile strength is determined experimentally under conditions of alternating stresses under a certain type of load change cycle.
Obtaining strength characteristics
Strength characteristics at increased deformation rates are obtained using pneumo-hydraulic devices. The action of impacts and explosions is used for deformation at very high speeds. If the relative strain rate is less than 104 s-1, the strain curve is determined in quasi-static tests, which ensure a uniform stress state throughout the entire volume of the working part of the sample, while maintaining this speed. The effect of strain rate is insignificant for brittle materials of high strength and increases with increasing their ductility.
The presence of structural and technological stress concentrators (holes, sharp transitions in shape, places with a non-uniform material structure) significantly reduces the dynamic strength of structural elements.